Embryology Biology 441 Spring 2010 Albert Harris
�������������������������������������������������More Review Questions for Second Examination:
Describe & name the kind of symmetry or combination of symmetries of any letter of the alphabet, or other geometric pattern (for example, Z has two-fold rotational symmetry, A has one plane of reflection symmetry, * has six planes of reflection symmetry), and also the symmetries of embryos at different stages of development.
Which differentiated cell types are capable of crawling locomotion? Describe the forces that propel crawling tissue cells. Will body cells move preferentially onto (and into) more materials to which they are more adhesive? What is meant by "Haptotaxis"? Is this preferential adhesive movement onto more adhesive materials because cells are physically pulled by the physical process of forming more or larger adhesions? (Hint: No). By what method were the locations and directions of this force discovered? What is meant by chemotaxis? Can you think of some embryonic events that could be controlled by haptotaxis?
According to what hypothesis is haptotaxis the cause of gastrulation, neurulation and sorting out by dissociated and randomly mixed cells? Hint: this hypothesis is usually explained in terms of thermodynamics. Among authors of textbooks, name two who are absolutely convinced of the truth of this hypothesis. What German biologist with a famous last name has reported discoveries that he believes disprove this hypothesis?
Two possible reasons why a mass of dissociated cells might behave as if it had a contractile layer at its surface are (1) ____ and (2) _____.
How does the "Theory of Positional Information" interpret embryonic regulation as evidence for control of cell differentiation and shape formation by linear diffusion gradients of chemicals called "morphogens"? Are there other ways to interpret embryonic regulation? What is a prepattern?
* Try to invent hypotheses to explain the following facts: Most non-cancerous cells become shaped differently when crawling on less adhesive surfaces, as compared with how they behave on glass or other sticky surfaces. On less adhesive surfaces, the normal cells mimic shapes and movements of cancer cells. ** Cancer can be induced by several kinds of solid materials, including some forms of asbestos, if these solids become imbedded in your tissues. ** Hypothesize the mechanism. * Which is more true: a) That cells pull themselves by adhesions to external materials, or b) That cells are pulled by formation of adhesions (that expansion of adhesions exerts a pulling force)? (Hint (a) is more true)
The "Differential Adhesion Hypothesis" explains sorting out of differentiated cells from one another by assuming which of the two alternatives in the preceding question? Does the author of our textbook seem to accept the Differential Adhesion Hypothesis as having been proven true? (Hint, he sure does!) Compare the opinions of Hans Holtfreter and J. P. Trinkaus about this ("DAH") theory. Compare their opinions about complicated theories, in general, as applied to embryology. In terms of the "DAH" what would it mean about differences between normal and cancer cells if they could sort out from each other, starting with random mixtures, and the cancer cells tended to wind up selectively on the outside surface of the masses of cells? True or false: The anatomy of every kind of multicellular animal consists of some particular geometric arrangement of differentiated cells? (Hint, yes, although it's a little more complicated than just arrangement of cells; collagen and other extracellular materials also have to get arranged, and epithelial cells have to get the correct orientations.
Review of Curvature, Stress (=tension) and Symmetry Concepts Important to Embryology
Those questions in ITALICS are more difficult than you need to understand for the exam
1) What are some examples of quantitative variables that are scalars? What is special about vectors, and variables that are vectors. What is the definition of curvature? (for example, the curvature of a line). Compare the curvature of a small circle, a large circle, and a straight line. [answer: small circles have large curvatures, large circles have smaller curvatures, and straight lines have zero curvature.] Compare the curvatures of a small sphere, a large sphere, a flat plane, a hen's egg, and a saddle.
2) The surface of what shape has the same (non-zero) curvature in every direction at every point on its surface? A bigger circle has a (larger? smaller? the same? you can't necessarily tell?) curvature than a smaller circle?
3) Consider an ordinary light bulb, that looks like this: 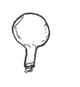
-
a) What parts of its surface have the same curvature in all directions?
b) What parts of its surface have zero curvature in two (exactly opposite) directions?
c) What parts of its surface consist of "saddle points" whose maximum curvature is positive in one direction, but which has a negative curvature in the perpendicular direction (or axis)?
-
a) The embryos of most species, from early oogenesis until after gastrulation begins?
b) The aggregated mass of Dictyostelium amoebae, before it forms a slug?
c) An early limb bud, before it develops its asymmetries (in its ant.-post. and dorso-ventral axes?
d) Soap bubbles and water drops
e) Dead cells
6) a) If you weaken the tension in part of the surface of a balloon (for example, if you put some chemical on it that reacted with the rubber, and weakened it), then how would that change the size and shape of the balloon? b) What if you somehow made part of the rubber more contractile?
7) *If the tensions in the rubber of an inflated balloon (at most locations on the surface) are twice as strong in one direction as they are in the perpendicular direction (that is, in the direction perpendicular to that in which they are stronger) then predict the shape of the balloon.
8) When an artery bursts because of excess blood pressure, can you predict the direction of the long axis of the rip in the artery wall?
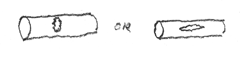
9) The smooth muscles and collagen fibers that form most of the walls of your arteries are oriented with their long axes pointed mostly in the circumferential drection, relative to the long axis of the artery. Why? How?
10) Imagine that some disease caused these smooth muscles and collagen fibers to become reoriented, equally in all directions parallel to the surface of the artery: What would happen, and why?
11) If an organ (or an organism) is shaped by two (or more?) exactly counter-balanced forces (of any kind), then if both forces have spherical symmetry (the same strength in all directions), then what shape will the organ (or organism) be, whose shape is caused by these forces?
12) If this organ (or organism) then spontaneously changes to some shape with a different symmetry, then can you deduce from that something about the directional symmetry of whatever forces cause this spontaneous change in shape? (hint: yes; those new forces have to be asymmetrical)
13) The brain and spinal cord start out as a hollow, liquid filled cylinder. Then the brain expands greatly relative to the spinal cord, with some parts (mid-brain, forebrain, eye-cup) bulging outward more than others. How will brain shape be controlled by weakening of tension in some directions relative to others?
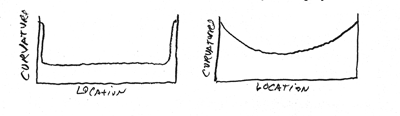
16) Consider the following two graphs of amounts of curvature in two perpendicular directions on the surface of a hollow ball. Figure out what game is played with this ball!
17) Try to visualize a solid shape whose surface has zero curvature in one direction, and whose curvature in the direction perpendicular to this is some constant amount. This solid shape is called a what? Can you draw one? And draw arrows in different directions on its surface, with the length of each arrow being proportional to the amount of curvature in that direction.
18) What is meant by saddle-points, in terms of the curvature of a surface? For the following objects, do any parts of their surfaces consist of saddle points? And please draw those parts (if any) that do consist of saddle points, for a) A cell, late in mitotic division? b) An ordinary light bulb? c) The surface of one of your hands? d) A branch-point in a blood vessel? e) The surface of the brain in early development? f) The surface of a teleost fish egg at the one-cell stage? g) A slime mold slug?
19) At saddle points, are there certain directions in which the local curvature is zero in two directions? From what logical argument do you reach this conclusion?

20) If you dip a ring in a soap solution in water, then a continuous soap film will remain, stretched across the ring: In terms of the forces that cause its shape, then why is this soap film flat? What curvatures does a flat surface have?
21) If you had a circle or ring made out of metal, and bent parts of this metal ring so that they were not all in the same plane, and you dipped this in a soap solution, and took it out, and a continuous sheet of soap formed across the interior of this bent ring, then this soap film will NOT be flat. However, at each point, if you measure the curvatures in any two perpendicular directions, and add these two curvatures together, the sum of these curvatures will be what constant amount?
22) On this non-flat soap film, are any of the points saddle-points, in the sense that curvatures in perpendicular directions are "negative" relative to each other?
23) No matter how complicated or irregularly you bend the edge of such a ring, the soap film inside it will always be shaped so that what (?) rules are obeyed by the curvatures at each point on the film.
24) If you stretch a thin rubber sheet across such an irregularly bent ring, then what rules govern the local curvatures at each point? (as compared with the curvature in the perpendicular direction at that same point? as compared with the curvatures at other points on the same rubber surface?)
25) I have drawn an ellipse. ![]()
Can you draw a graph of the relative amounts of curvature of the ellipse at all the different points around its periphery?
26) I have drawn an eye-ball in side view. 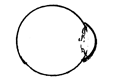
Can you draw a graph of the relative amounts of curvature at each point around its surface, as seen in this drawing?
-
a) The curvatures along the direction parallel to the sheet of paper?
b) The curvatures at each of the same points in the direction perpendicular to the paper?
28) If you have two soap bubbles, stuck together so that there is only one soap film along the side where they touch, then if one soap bubble has a smaller diameter than the other, the side of the smaller bubble will always bulge into the side of the bigger bubble. Can you explain this in terms of an equation relating P, T and C?
29) In this situation, there are effectively three curved soap films, each of which has a different surface curvature (C1, C2 and C3), and within each of the three the curvatures are the same in all directions at each point. Can you figure out a simple algebraic equation that relates these three curvatures to each other, and to the pressures inside the two bubbles (P1 and P2)?
30) Two rubber balloons, sharing a common side, would not obey this same equation, unless the tensions in different parts of their rubber surfaces happened to have what properties?
31) In a case of two such rubber balloons sharing a common side, if one balloon had a smaller diameter, but did NOT bulge with a convex surface toward the larger balloon, then what could you conclude about the pressures in the two balloons, and how much force is required to stretch different parts of their surfaces in different directions?
32) If you had a balloon that is NOT spherical when inflated (maybe shaped like Mickey Mouse's head) could you figure out the different amounts of tension in different parts of its surface based on observing or measuring the curvatures at different places on its surface?
33) In a cylindrical pipe, or hose, or balloon, only the tensions in the circumferential direction contribute to resisting outward pressure. Do you see why that is true? Hint: what does it have to do with the amounts of curvature in the longitudinal compared with the circumferential direction?
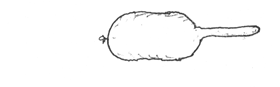
34) If you have a balloon that becomes cylindrical when inflated (but of course has hemispherical ends) sort of like a hot dog, then when this balloon is only half-inflated it will have the shape drawn below. What are the curvatures in different parts of a surface with this shape?
Are there differences in air pressure from one part of such a balloon and another part?
When you push in on different parts of this balloon's surface why does it feel like the air pressure is much larger in the more inflated part?
In which parts is the rubber stretch more tightly?
Try to draw a map of the differences in amount and direction of tension of the rubber that the balloon is made out of in different parts of this partially-inflated balloon.
----------- More Review questions Saturday Feb 27, 2010
1) Quantitative variables that only have an amount at each location are called s----- variables. Osmotic pressure is one example; what are at least two other examples.
2) Vectors are variable that have a -------- and also an amount, at each location in space (although this amount may be zero at a given point, in which case, there is no direction, either.
3) The amount of curvature of a line drawn on a plane is defined as the rate of change of what, per distance along a line?
4) A circle has -------- curvature. A big circle has ---- curvature than a small circle.
5) A sphere has how much curvature in each direction, parallel to its surface.
6) Sketch the shape of a light bulb, and describe the directional variations of its surface curvature, on different parts of its surface. For example, are there some parts that have a constant curvature, in all directions? Are there other parts in which the curvature in one direction is zero, but there is a curvature in all but that one direction? Find the small area in which curvature is positive in some directions, but negative in other directions at those same points. Such places are known as saddle points.
7) Are there saddle points on surfaces shaped like a Dictyostelium slug? Shaped like a hand or a glove, a doughnut, or an eyeball with its bulging cornea.
8) Describe the directional curvatures on the surface of a football (American football; a soccer ball is a sphere.)
9) S----- includes both compressive s------ and tensile s-----. Within a continuous piece of material (a wall, the surface of a balloon, a wooden board holding up weights, an embryo), this variable has an amount in each direction, so that it is another example of a T----- variable.
10) In the wall of a water-filled pipe, including any blood vessel, or kidney duct, the curvature is ------- in one direction, but in directions perpendicular to that is ----- in small blood vessels and ----- in large blood vessels.
11) The pressure difference between one side of a flexible layer and the other (the inside versus the outside of a bubble or balloon) is what, in terms of T and t (tensile stresses in perpendicular directions) and C and c (surface curvatures in those same directions.
12) If you make a bent wire ring, dip it into soapy water, and create a soap film, then because there is zero pressure difference between the two sides, and because T (tensile stress = tension in a soap film automatically adjusts itself so that it is the same at every direction at every location, therefore C + c = what? (the sum of curvatures in perpendicular directions equals what?)
13) If you had a soap bubble, supported between two wire rings (which could be square, rectangular, or any shape, including irregular shapes), then P=T (C+c), which means what about how curvatures will vary relative to each other, from one point to another. Do perpendicular curvatures at each point vary independently of each other? Or do the vary inversely? Or does one vary and the other stay constant? Where one gets big, how does the other change? Or maybe C times c is some constant?
*14) Suppose that T became weaker where curvature was smaller, how would this change the shapes formed? Suppose T became stronger where C was smaller: what then.
*15) In order for genes to cause all sorts of different anatomical shapes to form, what shapes can be created by controlling local variations of curvature?
*16) For the purpose of creating shapes (and making them stable), which variable would you rather control directly, and let the other two take care of themselves: Pressures, Curvatures, or Stress?
* When humans build something out of more-or-less flexible materials (tents, domes, suspension bridges, blimps, dirigibles, airplanes, dams [please suggest some more], which of these variables (P, C or T) do we impose arbitrarily on the construction materials, and which do we let be determined as secondary results?
* Embryonic development creates shapes by controlling which of these variables, and letting which other variables change as secondary results? When cell traction is used?
When water is pumped into closed cavities (the blastocoel, the neurocoel, the eyeball?) When osmotic pressures are created? When cells grow?
** Who tends to dictate the Cs and let the Ts and Ps take care of themselves?
** Who tends to dictate the Ts and Ps, and lets the Cs take care of themselves?
*** What about D'Arcy Thompson? Which did he think varied secondarily, as results of controlling which?
** Do you yourself have a preference? Which do you think embryos do? Which approach is more practical? Which is more economical? Which is more elegant?
* When people explain organ shape by "growth", they are tacitly assuming which of these causal sequences between P, T and C.
* Imagine starting with a spherical balloon, and sticking duct tape onto certain parts of its surface, and maybe gluing rubber bands to parts of its surface, with the purpose of making it develop particular arbitrary shapes when inflated. Could you make it inflate to the shape of Mickey Mouse? Could you make it inflate to the shape of a light bulb? What about the shapes of developing bones? What about the shape of the heart, or the kidneys? Think of some other organ, organism, or cell shapes.
��������������������������������������������������������������������������������